Electronic components are classed into either being Passive devices or Active devices. Active devices are different from passive devices. These devices are capable of changing their operational performance, may deliver power to the circuit, and can perform interesting mathematical functions. While a device that does not require a source of energy for its operation.

What are Active Devices?
An active device is any type of circuit component with the ability to electrically control electron flow (electricity controlling electricity). In order for a circuit to be properly called electronic, it must contain at least one active device. Active devices include, but are not limited to, vacuum tubes, transistors, silicon-controlled rectifiers (SCRs), and TRIACs.
All active devices control the flow of electrons through them. Some active devices allow a voltage to control this current while other active devices allow another current to do the job. Devices utilizing a static voltage as the controlling signal are, not surprisingly, called voltage-controlled devices. Devices working on the principle of one current controlling another current are known as current-controlled devices. For the record, vacuum tubes are voltage-controlled devices while transistors are made as either voltage-controlled or current controlled types. The first type of transistor successfully demonstrated was a current-controlled device.
What are Passive Devices?
Components incapable of controlling current by means of another electrical signal are called passive devices. Resistors, capacitors, inductors, transformers, and even diodes are all considered passive devices.
Passive devices are the resistors, capacitors, and inductors required to build electronic hardware. They always have a gain less than one, thus they can not oscillate or amplify a signal. A combination of passive components can multiply a signal by values less than one, they can shift the phase of a signal, they can reject a signal because it is not made up of the correct frequencies, they can control complex circuits, but they can not multiply by more than one because they lack gain.
Diodes
Diodes are basically a one-way valve for electrical current. They let it flow in one direction (from positive to negative) and not in the other direction. Most diodes are similar in appearance to a resistor and will have a painted line on one end showing the direction or flow (white side is negative). If the negative side is on the negative end of the circuit, current will flow. If the negative is on the positive side of the circuit no current will flow. More on diodes in later sections.

Integrated Circuits
Integrated Circuits, or ICs, are complex circuits inside one simple package. Silicon and metals are used to simulate resistors, capacitors, transistors, etc. It is a space saving miracle. These components come in a wide variety of packages and sizes. You can tell them by their "monolithic shape" that has a ton of "pins" coming out of them. Their applications are as varied as their packages. It can be a simple timer, to a complex logic circuit, or even a microcontroller (microprocessor with a few added functions) with erasable memory built inside.

Transistors
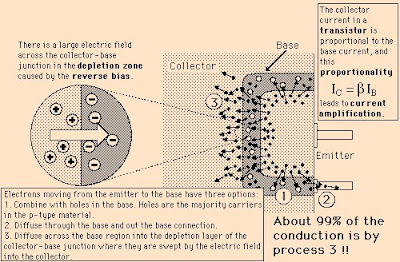
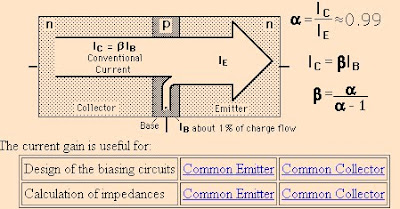
A transistor is a semiconductor device, commonly used as an amplifier or an electrically controlled switch. The transistor is the fundamental building block of the circuitry in computers, cellular phones, and all other modern electronic devices.
Because of its fast response and accuracy, the transistor is used in a wide variety of digital and analog functions, including amplification, switching, voltage regulation, signal modulation, and oscillators. Transistors may be packaged individually or as part of an integrated circuit, some with over a billion transistors in a very small area - part of a trend of increasing transistor density known as Moore's Law.
Transistor stands for transit resistor, the temporary name, now permanent, that the inventors gave it. These semidconductors control the electrical current flowing between two terminals by applying voltage to a third terminal. You now have a minature switch, presenting either a freeway to electrons or a brick wall to them, depending on whether a signal voltage exists. Bulky mechanical relays that used to switch calls, like the crossbar shown above, could now be replaced with transistors. There's more.
Transistors amplify when built into a proper circuit. A weak signal can be boosted tremendously. Let's say you have ten watts flowing into one side of the transistor. Your current stops because silicon normally isn't a good conductor. You now introduce a signal into the middle of the transistor, say, at one watt. That changes the transistor's internal crystalline structure, causing the silicon to go from an insulator to a conductor. It now allows the larger current to go through, picking up your weak signal along the way, impressing it on the larger voltage. Your one watt signal is now a ten watt signal.
Transistors use the properties of semi-conductors, seemingly innocuous materials like geranium and now mostly silicon. Materials like silver and copper conduct electricity well. Rubber and porcelain conduct electricity poorly. The difference between electrical conductors and insulators is their molecular structure, the stuff that makes them up. Weight, size, or shape doesn't matter, it's how tightly the material holds on to its electrons, preventing them from freely flowing through its atoms.
First Transistor

Active Versus Passive Devices in the News
Seven Steps to Successful Analog-to-Digital Signal Conversion
Understand how to balance gain blocks and noise, and perform noise calculations for proper signal conditioning Signal processing - Business - Technology - Electronics - Integrated circuit
Class D Speaker Amplifier offers automatic level control.
Designed for battery-operated portable devices, 2.2 W MAX98500 integrates boost converter to provide constant output power. Class D amplifier is equipped with battery-tracking Automatic Level Control circuit that limits max output swing as supply voltage drops. ALC helps to avoid clipping and prevents battery voltage from collapsing. Housed in 2.1 x 2.1 mm WLP, MAX98500 accepts 2.5-5.5 V supply ...
Rohde & Schwarz find scope for expansion
Rohde & Schwarz is today launching its first dedicated oscilloscopes. It has developed its own ASICs and A to D converters to support the new design which is described as 20 times faster than existing devices. Oscilloscope - Technology - Theory of Measurements - Electronics - Integrated circuit